Sharpness of Games and Players
Extending the sharpness score from individual positions to whole games
I've written quite a bit about sharpness in the past. But until now I've mostly looked at the sharpness of individual positions instead of whole games or even a larger number of games.
In a previous post, I calculated the average change in the sharpness for individual players. I was quite happy with the concept of looking at the change in sharpness - instead of the overall sharpness of a position - since this allows one to isolate the sharpness a single player.
But I wasn't too happy with the average of that score, since an average can hide a lot of information. The results can also be skewed by long endgames, where one doesn't have many opportunities to dictate the sharpness of the play.
In this post, I'll try to address some of the shortcomings of my previous attempts when looking at the sharpness of whole games.
Sharpness of a Game
First of all, I decided to look at the sharpness of a single game.
I did this by looking at the change of sharpness after each move by White and Black. So if White plays a move which makes the position sharper, the sharpness change is positive for this move. Conversely, if they make a move which decreases the sharpness, like declining an option to complicate the game, the sharpness change will be negative.
Let's look at some examples to see how these graphs look in practice.
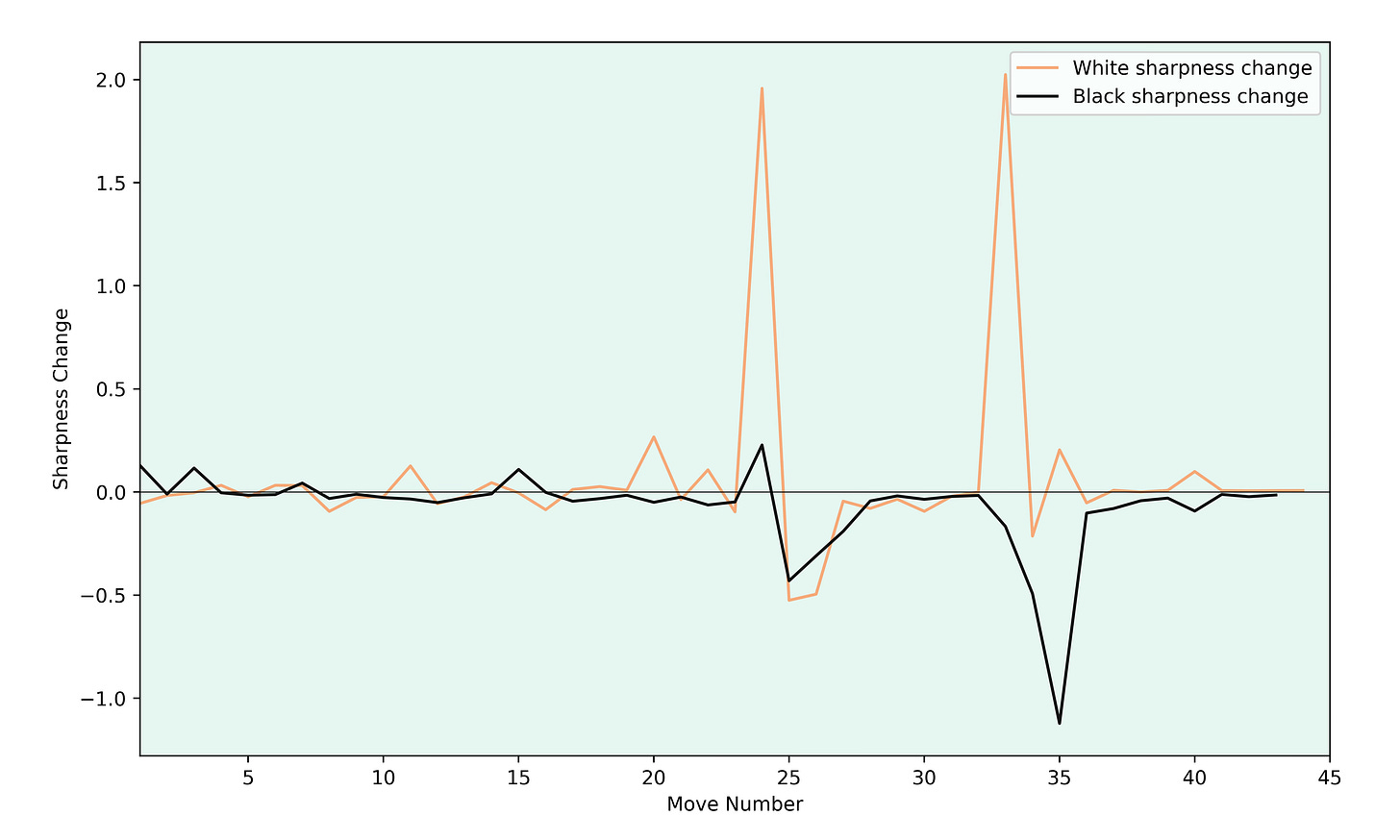
I first looked at the game Kasparov-Topalov, 1999:
There are two big spikes in the sharpness change of Kasparov's play. The first one is his rook sacrifice on move 24:
Here Kasparov played 24.Rxd4!!
The second spike is a bit more curious since it's for White's 33rd move:
Kasparov played 33.c3! which is a great move but at least to me it's much less striking than 24.Rxd4!!
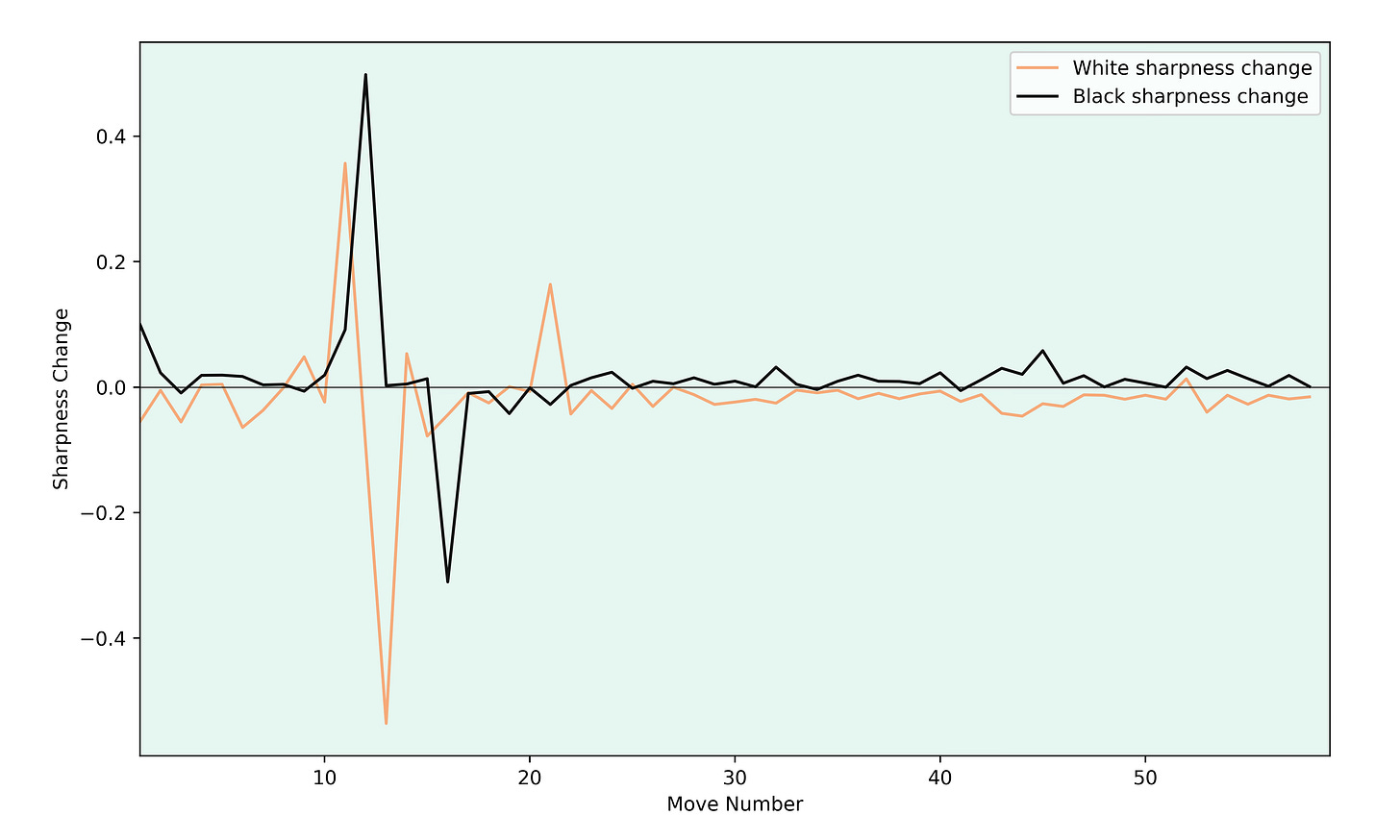
I was also interested in how these graphs look if the players take risks early on in the game. I decided to look at game 9 from the Tal-Botvinnik match in 1960. The graph looks much more exciting early on:
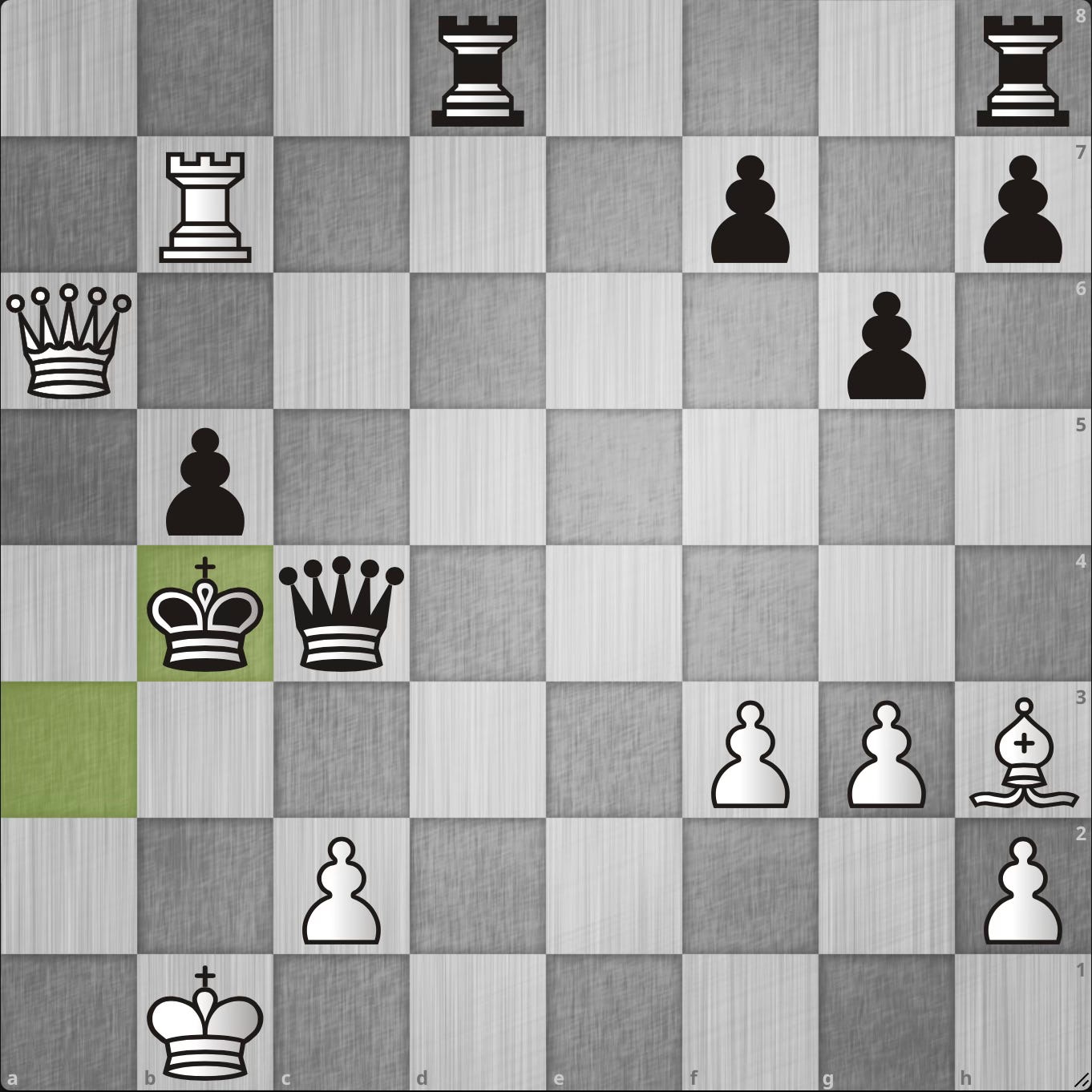
It all starts in this position.
Here Tal as White played 11.Nxe6 which is the first peak in sharpness for White. Then after 11...fxe6 12.Bxe6, Botvinnik played 12...Qc7 which is the big sharpness spike for Black in the graph above. Tal played 13.Re1 which the engines dislike and which also leads to a big drop in sharpness.
I think that these graphs are very interesting, since they give a different insight into a game than just the evaluation. However, it’s important to keep in mind that the sharpness isn’t completely independent of the evaluation. So some spikes in the graphs might be caused by mistakes instead of moves with the intention to complicate the game.
Sharpness for Players
I'd also like to measure the sharpness of players over many games instead of just one.
As mentioned in the introduction, I looked at the average sharpness change for players in the past. But I was never quite happy with this because the average hides a lot of information and is effect by the length of the game.
Since I liked the breakdown of the sharpness change per move, I decided to do the same with multiple games of the same player. For each player I calculated the average of the sharpness change for each move. So the sharpness change for move 5 in the graph is the average of the sharpness change of all fifth moves a player played in the games looked at.
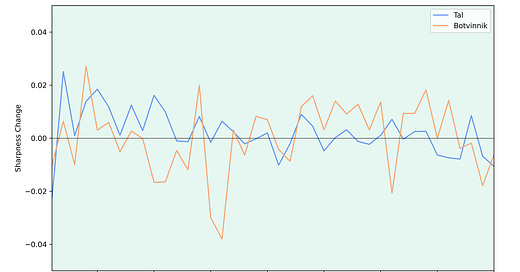
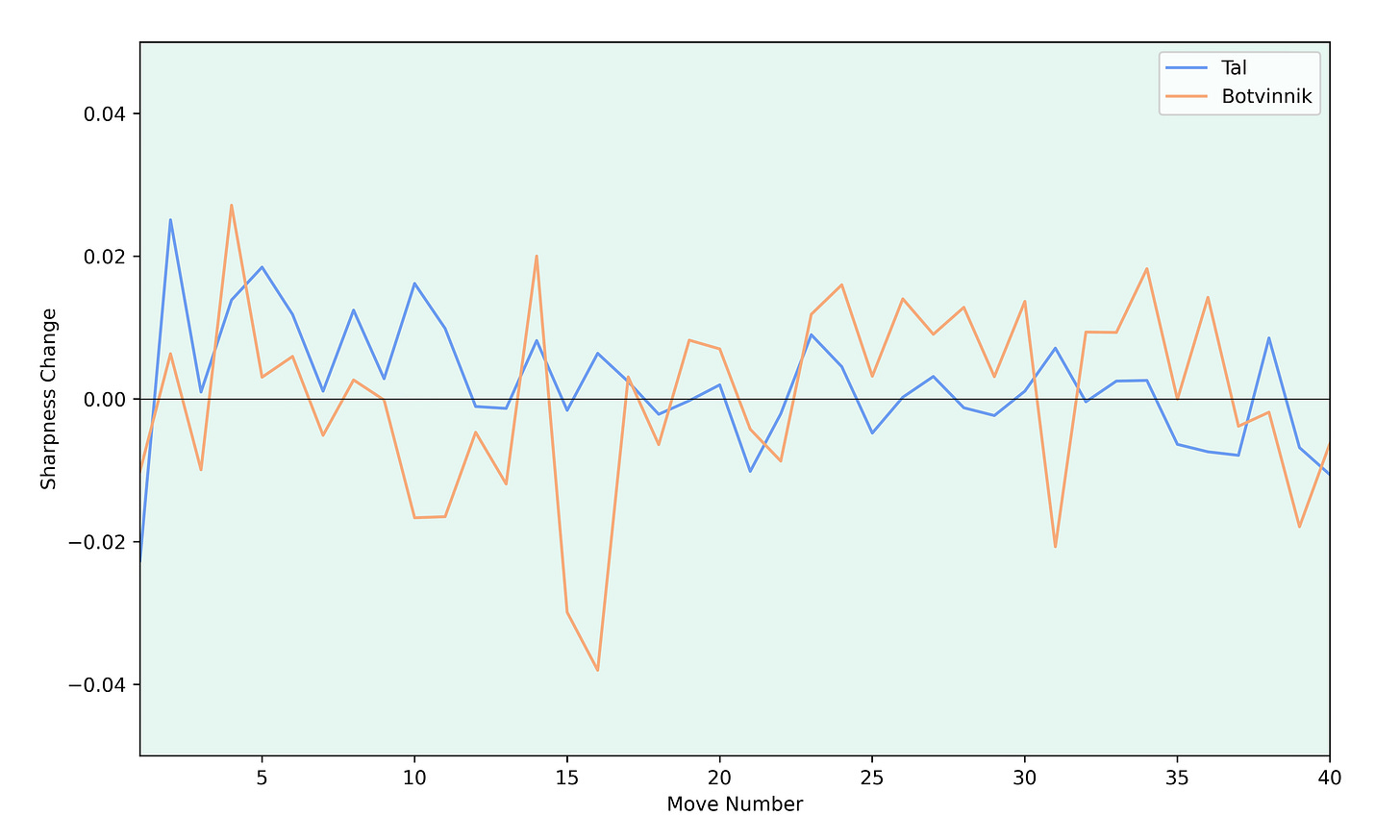
As an example, I decided to look at the games of Botvinnik and Tal from 1959 to 1961:
I honestly expected a bigger difference between the two players. Tal's sharpness change is higher early on in the game, which might suggest that he aimed for sharper positions right out of the opening. Botvinnik's sharpness change is higher later on which could mean that he tried to increase the tension in the middle game after a quieter opening.
But all this is speculation since it's difficult to exactly say why the graphs look the way they do.
Future Plans
I'm quite happy with the sharpness change for individual games. It might be especially useful when also looking at an evaluation graph of the game to see how the sharpness relates to the objective evaluation.
However, I'm not completely happy with the sharpness change for multiple games. Maybe it's just difficult to encapsulate the sharpness of a player in a single number which is calculated from all their games. After all, everyone has quieter and sharper games, so every score will be probably closer together than one would intuitively expect.
Let me know what you think about the way I approached these questions and if you have any additional ideas for measuring the sharpness of a player, write a comment.